Matrices & Determinants is an important topic that is asked in NDA, Air Force Group X & Y Exam. It is one of the major topic that is asked in the examination. We are also Sharing a PDF for your reference.
Matrices and Determinants Notes
1. Matrices
A matrix is an arrangement of elements (numbers, mathematical expressions) in a rectangular arrangement along ‘m’ number of rows and “n”: number of columns.
1.1 Types of Matrices
(a) Row Matrix
A matrix in which all elements are arranged in a single row.
(b) Column Matrix
A matrix in which all elements are arranged in a single column.
(c) Square Matrix
A matrix in which all elements are arranged in an equal number of rows and columns.
The elements in a square matrix for which the number of the row is equal to the number of the column is called the leading diagonal or the principle diagonal of the matrix.
The sum of the principle diagonal elements of a square matrix is called the trace of the matrix.
(d) Idempotent Matrix
A square matrix is called idempotent if the product of the matrix with itself results in the same matrix.
(e) Diagonal Matrix
A matrix in which all elements except those in the leading diagonal are zero.
(f) Scalar Matrix
A diagonal matrix in which all elements of the leading diagonal are equal.
(g) Unit Matrix
A diagonal matrix in which all elements of the leading diagonal are an equal one.
(h) Null Matrix
A matrix in which all elements are zero.
(i) Symmetric Matrix
A matrix in which the element of the ith row and jth column is equal to the element of the jth row and ith column.
(j) Skew-symmetric Matrix
A matrix in which the element of the ith row and jth column is equal to the negative of the element of the jth row and ith column, such that all elements of the principle diagonal are zero.
(k) Triangular Matrix
- Upper triangular Matrix: A matrix in which all elements below the principle diagonal are zero.
- Lower triangular Matrix: A matrix in which all elements above the principle diagonal are zero.
1.2 Algebra of Matrices
(a) Elementary Transformations of a matrix
(i)Interchange of rows/columns
(ii) Multiplication of a row or column by a non-zero number.
(iii) The addition/subtraction of a constant multiple of the elements of any row (or column) to the corresponding elements of any other row (or column)
(b) Equivalent Matrices: Two matrices are said to be equivalent if one of the matrices can be obtained by elementary transformations on the other.
(c) Equal Matrices: Two matrices are said to be equal if and only if
(i) The order of the matrices is same
(ii) The corresponding elements of matrices are equal
(d) Addition / Subtraction of Matrices: Two matrices can be added/subtracted if and only if the order of the matrices is same. The resultant matrix is the addition/subtraction of the corresponding elements.
(e) Multiplication of a matrix and a scalar: When a scalar is multiplied to a matrix, the product is the scalar-multiplied to each of the corresponding elements of the matrix.
(f) Multiplication of two matrices: Two matrices can be multiplied if and only if the number of rows in the first matrix is equal to the number of columns in the second matrix.
Note: It is not necessary that if two matrices X and Y are multiplied then XY =YX.
(g) Transpose of a matrix
The transpose of a given matrix is the matrix obtained by interchanging the elements of rows and columns.
For a Symmetric matrix: X = XT
For a Skew-symmetric matrix: X= - XT
Note:
(i) The transpose of a product of the two matrices is taken in reverse order i.e. (XY)T=YTXT
(ii) Any square matrix can be expressed as the sum of a symmetric matrix and skew-symmetric matrix.
Here, 
is a symmetric matrix while 
is a skew-symmetric matrix.
2. Determinants
A determinant for a given matrix exists only if it is a square matrix. It results in a single number or mathematical expression. It is denoted as |A| or.
It is evaluated as the sum of the products of elements of any row (or column) with its corresponding cofactor. For example, for matrix X of order 3,
2.1 Properties of Determinants
1. A determinant remains unaltered in its numerical value if the rows and columns are interchanged.
2. If two parallel rows (or columns) are interchanged, then the determinant retains its numerical value but changes its sign.
3. A determinant is zero if any two parallel rows (or columns) are proportional.
4. If each element of a row (or column is multiplied by the same factor, the whole determinant is multiplied by the same factor.
5. If each element of a determinant contains ‘n’ terms, then the determinant can be expressed as the sum of ‘n’ determinants.
6. A determinant remains unaltered in its numerical value if the rows and columns are interchanged.
7. If two parallel rows (or columns) are interchanged, then the determinant retains its numerical value but changes its sign.
8. A determinant is zero if any two parallel rows (or columns) are proportional.
9. If each element of a row (or column is multiplied by the same factor, the whole determinant is multiplied by the same factor.
10. If each element of a determinant contains ‘n’ terms, then the determinant can be expressed as the sum of ‘n’ determinants.
2.2 Cofactors, Adjoint and Inverse of a Matrix
Note: If the determinant formed by the cofactors of the corresponding elements of a matrix is equal to the square of the determinant of the matrix, then.
(a) Singular matrix
A singular matrix is a matrix whose determinant is zero.
(b) Cofactor of any element of the determinant
The cofactor is the determinant obtained by removing the row and column which intersect at that element and with a sign obtained as the (-1)i+j for the cofactor of the element xij .
For example, in a determinant 
, the cofactor of a11 is (-1)1+1 a22 = a22 and the cofactor of a12 is (-1)1+2 a21 = - a21.
(c) Adjoint of a matrix
The adjoint of a matrix is the transpose of the matrix formed by taking the cofactors of each element to form a matrix.
It is denoted by adj A for a matrix A.
(d) Properties of adjoint of matrix
If X is a square matrix of order n
(i) |adj X| = |X|n-1
(ii) adj (adj A) = |A|n-2 A,
(iii) |adj (adj A) | = |A|(n-2)(n-2)
(iv) adj (AB) = (adj B) (adj A)
(v) adj (kA) = kn-1 (adj A), k is any scalar
(vi) adj AT = (adj A)T
3. Inverse of a Matrix
The inverse of a non-singular matrix is a matrix which when multiplied to the original matrix results in an identity matrix.
Using adjoint, the inverse of a matrix can be evaluated as
4. Area of triangles using determinants
If the coordinates of the vertices of a triangle are (x1, y1), (x2, y2) and (x3, y3) then the area of the triangle is expressed as
5. Test of consistency of linear equations
If A is the coefficient matrix, X is the variable matrix and B be the constant matrix, then the system of equations is represented as AX=B.
If 
, then the system has a unique solution.
If 
and
(a) 
there is no solution
(b)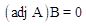 
there are infinite or no solutions.
6. Solution of Linear equations
6.1 Cramer’s Rule:
Consider the system of equations
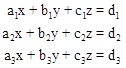
The determinant A is the determinant of the coefficient matrix. The determinant |Ax| is the determinant obtained by replacing the column of coefficients of x by the column of constant terms. Similarly, we have |Ay| and |Az|
Then, the solution of the system of equations is
x=|Ax| / |A|
y=|Ay| / |A|
z=|Az| / |A|
6.2 Matrix Inversion method
X=A-1B
Below is a PDF for the same:
Matrices & Determinants for NDA, Air Force Group X & Y Exam
More from us:
Important Study Notes for Defence Exams
Keep Learning
The Most Comprehensive Exam Prep App Go BYJU'S Exam Prep!
Comments
write a comment